Question

9. If h(x)=√x-3 +5, then for what value(s) of 10
x does h(x) = 3?

Answer 1

There are no values of x that make this equation true.

Explanation:

We know h(x)=
`√(x-3) +5`

So lets substitute h(x) for 3

3=
`√(x-3)+5`

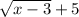
We are going to then subtract 5 from each side leaving us with

-2=
`√(x-3)`

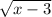
We then need to get rid of the square root, so we will square both sides. which gives us

4=x-3

We then need to subtract 3 from both sides which leaves us with

x=1

However, when you substitute 1 for x into the original equation, you get

h(x)=
`√(-2)+5`

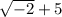
Which is not equal to 3

Therefore, there are no values of x that make this equation true.