Question

Find the slope of the line passing through the points (-4,6) and (3,6)

Slope:

Find the slope of the line passing through the points (-5,8) and (-5,-6)

Slope:
​

Answer 1

Required answer:

1. 0
2. Not Defined

Detailed explanation:

To find the slope of the line, given that it passes through two points, use the formula:

`\bf{m=(y_2-y_1)/(x_2-x_1)}`

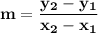
Where:

• m = slope

Plug in the data:

`\bf{m=(6-6)/(3-(-4))=(0)/(3+4)=(0)/(7)=\boxed{\bf{0}}`

- - - - - - - - - - - - - - - - - - - - - -

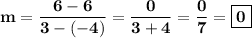
Given the pair of points: (-5,8) and (-5,-6), plug them into the slope formula:

`\bf{m=(y_2-y_1)/(x_2-x_1)}`

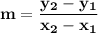
Evaluate:

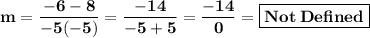
`\bf{m=(-6-8)/(-5(-5))=(-14)/(-5+5)=(-14)/(0)=\boxed{\bf{Not\:De fined}}`

- - - - - - - - - - - - - - - - - - - - - - -

Answer 2

Answer:
1. 0
2. Undefined

Step-by-step explanation:
To find the slope of the two points, you first have to subtract the y1 from the y2 (6-6). Then, you do the same thing with the x1, and the x2 (3-(-4). Your slope is 0/7. 0/7 simplified is 0, since the 0 is the numerator, not the denominator.

Next, you can do the same thing with the second slopes. First is -6-8, which is the numerator, and then do -5-(-5), which is the denominator. Your answer comes out to -14/0. If the denominator is 0, the answer is will always be undefined.

Hope that helped!!