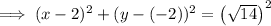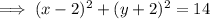Answer:
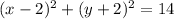
Explanation:
Equation of a circle

where:
- (a, b) is the center of the circle.
- r is the radius of the circle.
Given equation:
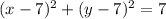
Therefore:
- center = (7, 7)
- radius = √7
Area of a circle
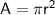
where:
Therefore, the area of a circle with radius √7 is:
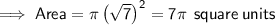
Therefore, a circle with twice the area would be:
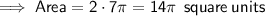
Therefore, its radius would be:
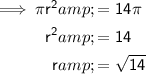
So the equation of a circle with a center at (2, -2) and a radius of √14 is: