Question

You have 2 positive numbers. One number is one-fifth of the other number. The difference between the two numbers is 308, find the numbers. PLEASE HELP

Answer 1

`77, 385`

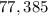
Explanation:

Start by writing the equations in symbolic form. I will represent one number with an x and the other with a y.

One number is one-fifth of the other number:

`x=(1)/(5)y`

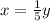
The difference between the two numbers is 308:

`y-x=308`

This difference is positive, therefore I am assuming that y is the larger number. In the first equation, I made x a part of y, so this should check out.

Now we have a system of equations:

`\left \{ {{x=(1)/(5)y } \atop {y-x=308}} \right.`

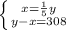
I will rewrite the first equation for simplicity:

`5x=y`

Now, I will substitute y from the first equation for y in the second equation:

`5x-x=308`

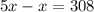
Simplify:

`4x=308\\x=77`

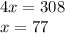
Now, use x to find y using the second equation:

`y-77=308\\y=385`

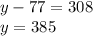
Now, I can check the solutions by plugging them into the first equation!

`x=(1)/(5)y\\ 77=(1)/(5)(385)\\77=77`

The solution is correct!