Answer: (-4-
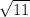 , -4+
, -4+
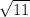 ) ==> B
) ==> B
Explanation:
x^2+8x+5<0
x^2+8x+16-11<0
(x+4)^2-11<0
(x+4)^2<11
x+4<
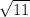
x<-4+
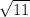
x+4>-
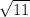
x>-4-
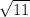
(-4-
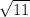 , -4+
, -4+
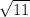 ) ==> B
) ==> B
Remember, the solution doesn't include the x values -4-
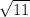 and -4+
and -4+
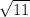 since if they were plugged in x^2+8x+5, the expression would equal 0. The expression is supposed to be LESS than 0, not equal to 0.
since if they were plugged in x^2+8x+5, the expression would equal 0. The expression is supposed to be LESS than 0, not equal to 0.