Answer:
∠XYZ = 72.9°
Explanation:
To find the size of an angle in a right-angled triangle, we can use the trigonometric ratios.
In the definitions of trigonometric ratios, the sides of a triangle are named relative to a particular angle, so that the side opposite to that angle is called 'opposite', the side beside the angle is called 'adjacent', and the side opposite to the 90° (right) angle is called the 'hypotenuse'.
In this triangle:
• opposite ⇒ XZ
• adjacent ⇒ XY
• hypotenuse ⇒ YZ
The trigonometric ratios are as follows:
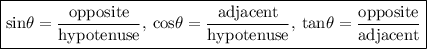 ,
,
where
 is the main angle.
is the main angle.
As we can see in the diagram, the lengths of the opposite and adjacent sides are given. Therefore, we have to use the formula for
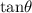 :
:
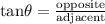
In this case,
 is angle XYZ.
is angle XYZ.
⇒
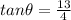
To solve for
 , take
, take
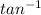 of both sides:
of both sides:
⇒
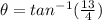
⇒

∴ Angle XYZ = 72.9°