Question

PLEASE I NEED HELP ASAP TO FILL IN THE BLANKS

Answer 1

(5x²+5x+7)-(5x²+5+7)= -5x²-14

Answer 2

`(2x^2+2x+1)-(7x^2+2x+15)=-5x^2-14`

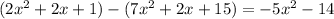
Explanation:

To explain how to approach this question, I am going to place letters in the blank spaces:

`(ax^2+bx+c)-(dx^2+ex+f)=-5x^2-14`

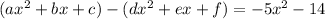
First, compare the coefficients of the x² terms on both sides of the equation:
`a - d = -5`

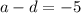
Therefore, we need to choose any two numbers in place of a and d whose difference is -5:

⇒ a = 2 and d = 7 as 2 - 7 = -5.

Similarly, upon comparing the coefficients of the x terms, we can see that there is no x term on the right side of the equation. Therefore, we need to choose numbers in place of b and e for which the difference is zero. So b and e should be the same number:

⇒ b = 2 and e = 2 as 2 - 2 = 0.

Finally, compare the constant term on both sides of the equation:
`c - f = -14`

Therefore, we need to choose any two numbers in place of c and f whose difference is -14:

⇒ c = 1 and f = 15 as 1 - 15 = -14.

Plug in the numbers in place of the letters on the left side of the equation:

`(2x^2+2x+1)-(7x^2+2x+15)=-5x^2-14`

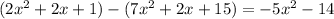
Check

Remove the brackets:

`2x^2+2x+1-7x^2-2x-15=-5x^2-14`

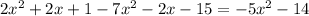
Collect like terms:

`2x^2-7x^2+2x-2x+1-15=-5x^2-14`

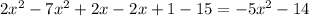
Combine like terms:

`-5x^2-14=-5x^2-14`

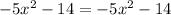
Hence proving that the left and right sides of the equation are the same.