Hi there,
I hope you and your family are staying safe and healthy!
Let's solve the equation
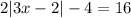
The first step here is to add 4 on both sides

We're not done yet! So now we need to divide both sides by 2
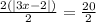
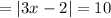
Now we need to solve for the absolute value
We know either
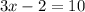 or
or
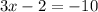
Therefore, there are two possibilities here.
Possibility 1

Possibility 2

Thus,
The answer is: x = 4 or x =
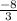
Happy to help! I wish you all the best this semester :)
-Garebear