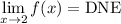Question

Pls help this is pretty urgent

Answer 1

(a) 4

(b) f(1)

(c) c = 2

Explanation:

Part (a)

Approach x = 2 from the left side of 2:

`\displaystyle \lim_(x \to 2^-)f(x)= \lim_(x \to 2^-)(x^2)=(2)^2=4`

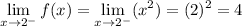
Part (b)

`\textsf{To find }\displaystyle \lim_(x \to 1)f(x):`

Approach x = 1 from the left side of 1:

`\displaystyle \lim_(x \to 1^-)f(x)= \lim_(x \to 1^-)(-x^2+2)=(-(1)^2+2)=1`

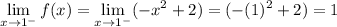
Approach x = 1 from the right side of 1:

`\displaystyle \lim_(x \to 1^+)f(x)= \lim_(x \to 1^+)(x^2)=(1)^2=1`

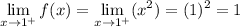
As the approach from the left and right go to the same y-value:

`\implies \displaystyle \lim_(x \to 1)f(x)=1`

`f(1)=2`

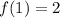
Therefore, as 2 > 1 then:

`\implies f(1) > \displaystyle \lim_(x \to 1)f(x)`

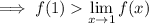
Part (c)

For a limit to exist at a point x = c, the right and left-hand limits must be equivalent at c.

From inspection of the graph, the limit for the value of c on the interval [0, 4] that does not exist is c = 2.

Approach x = 2 from the left side of 2:

`\displaystyle \lim_(x \to 2^-)f(x)= \lim_(x \to 2^-)(x^2)=(2)^2=4`

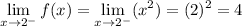
Approach x = 2 from the right side of 2:

`\displaystyle \lim_(x \to 2^+)f(x)= 3`

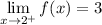
As the approach from the left and right do not go to the same y-value,

`\displaystyle \lim_(x \to 2)f(x)= \rm DNE`