Question

Which of the following is a true polynomial identity? NO LINKS!!!

​

Answer 1

`\textsf{b)} \quad x^2+y^2=(1)/(2)(x+y)^2+(1)/(2)(x-y)^2`

Step-by-step explanation:

Polynomial Identity

An equation that is always true for all possible values of the variable.

To find which of the given answer options is a true polynomial identity, expand the right side of each answer option and compare to the left side.

Answer option a

`\begin{aligned}(x+y)(x-y) & = x(x-y)+y(x-y)\\& = x^2-xy+xy-y^2\\& = x^2-y^2\end{aligned}`

`\textsf{As\: $x^2+y^2\\eq x^2-y^2$ \:for all values of $x$ and $y$,}`

`\textsf{this is \underline{not} a true polynomial identity.}`

Answer option b

`\begin{aligned}(1)/(2)(x+y)^2+(1)/(2)(x-y)^2 & = (1)/(2)(x+y)(x+y)+(1)/(2)(x-y)(x-y)\\& = (1)/(2)(x^2+2xy+y^2)+(1)/(2)(x^2-2xy+y^2)\\& = (1)/(2)x^2+xy+(1)/(2)y^2+(1)/(2)x^2-xy+(1)/(2)y^2\\& = (1)/(2)x^2+(1)/(2)x^2+xy-xy+(1)/(2)y^2+(1)/(2)y^2\\& = x^2+y^2\\\end{aligned}`

`\textsf{As\: $x^2+y^2=x^2+y^2$ \:for all values of $x$ and $y$,}`

`\textsf{this is a true polynomial identity.}`

Answer option c

`\begin{aligned}(x+y)^2 & = (x+y)(x+y)\\& = x(x+y)+y(x+y)\\& = x^2+xy+xy+y^2\\& = x^2+2xy+y^2\end{aligned}`

`\textsf{As\: $x^2+y^2\\eq x^2+2xy+y^2$ \:for all values of $x$ and $y,$}`

`\textsf{ this is \underline{not} a true polynomial identity.}`

Answer option d

`\begin{aligned}(1)/(2)(x+y)^2+(1)/(2)(x-y)^2 & = (1)/(2)(x+y)(x+y)+(1)/(2)(x-y)(x-y)\\& = (1)/(2)(x^2+2xy+y^2)+(1)/(2)(x^2-2xy+y^2)\\& = (1)/(2)x^2+xy+(1)/(2)y^2+(1)/(2)x^2-xy+(1)/(2)y^2\\& = (1)/(2)x^2+(1)/(2)x^2+xy-xy+(1)/(2)y^2+(1)/(2)y^2\\& = x^2+y^2\\\end{aligned}`

`\textsf{As\: $x^2-y^2=x^2+y^2$ \:for all values of $x$ and $y$,}`

`\textsf{this is \underline{not} a true polynomial identity.}`

Solution

Therefore, answer option b is the only true polynomial identity.

Answer 2

Answer: Choice B

`x^2+y^2 = (1)/(2)(x+y)^2 + (1)/(2)(x-y)^2`

===================================================

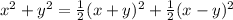
Step-by-step explanation:

Let's go through the answer choices to see which are true and which are false.

------------------------

Choice A is false because it should be

`x^2-y^2 = (x+y)(x-y)`

which is the difference of squares factoring rule.

However, the left hand side of choice A has a plus, and not a minus.

Consequently, this rules out choice D.

------------------------

Choice B is true

We can conclude this by eliminating the other answer choices as false, and by noticing the following:

`x^2+y^2 = (1)/(2)(x+y)^2 + (1)/(2)(x-y)^2\\\\x^2+y^2 = (1)/(2)(x^2+2xy+y^2)+(1)/(2)(x^2-2xy+y^2)\\\\x^2+y^2 = (1)/(2)x^2+xy+(1)/(2)y^2+(1)/(2)x^2-xy+(1)/(2)y^2\\\\x^2+y^2 = x^2+y^2`

This confirms why choice B is an identity. The equation is true for any real numbers x and y.

Let's look at an example. I'll pick x = 4 and y = 7

`x^2+y^2 = (1)/(2)(x+y)^2 + (1)/(2)(x-y)^2\\\\4^2+7^2 = (1)/(2)(4+7)^2 + (1)/(2)(4-7)^2\\\\16+49 = (1)/(2)(11)^2 + (1)/(2)(-3)^2\\\\65 = (1)/(2)(121) + (1)/(2)(9)\\\\65 = 60.5+4.5\\\\65 = 65\\\\`

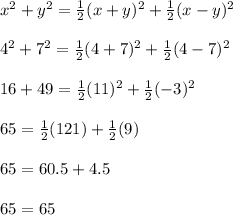
I'll let you try other (x,y) pairs of values. Keep in mind that using numeric examples like this is not a proof. The proof would be using algebra to expand and simplify the right hand side so it becomes the left hand side.

------------------------

Choice C is false. The right hand side
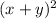`(x+y)^2` expands out to
`x^2+2xy+y^2`

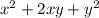
A common mistake students do is to forget about the 2xy when expanding out
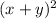`(x+y)^2`, which may lead to choice C being a slight trick answer.

If choice C said
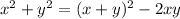`x^2+y^2 = (x+y)^2-2xy`, then we'd have a true identity

------------------------

Choice D is false. Refer to the formula I wrote in choice A.