Question

Graphing/Slopes (Easy points!!) (ASAP) (URGENT) (Please check my other questions)

​

Answer 1

The statement is incorrect because an inverse relationship between y and x would imply that the graph would be a downward curved graph whose slope changes rather than linear which has constant slope (see image).

Best to express this situation as:
There is a linear relationship between y and x with a negative constant slope. The keyword here is constant since the slope is the same in a linear relationship

Explanation:

An inverse relationship exists between y and x when y is proportional to the reciprocal of y

This would be expressed in the form
`y` ∝
`(1)/(x)`

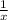
As x decreases , y increases or vice-versa. It is often expressed in equation form as
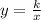`y=(k)/(x)` or
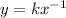`y = kx^(-1)` where k is a constant and whose graph is curved in nature. The slope is changing for the range of x values not constant as would be the case for a linear graph

All we can say about the data is that there is a linear relationship between y and x with a negative constant slope