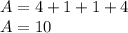Answer:
10
Explanation:
For my explanation I'll be referring to points labeled in the attached image.
Remember the formula for a triangle is:
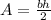
Where A is your area, b is your base, and h is your height.
Lets solve for the area of ABC
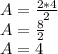
Now lets solve for BGF
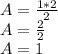
FHE has the same base and height as BGF, therefore its area is also 1.
Now solve for the square CDGH

Now find the sum of all areas