Question

The x intercepts of the function f(x) = 2x(x-5)^2(x+4)^3
are​

Answer 1

x- intercepts (0,0) , (5,0) , (-4,0)

y- intercepts (0,0)

Hope this helped

Answer 2

Answer:

`\boxed{\sf x- intercepts = 0 , 5 \ and \ -4}`

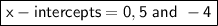
Explanation:

A function is given to us and we need to find the x Intercepts of the graph of the given function . The function is ,

`\sf \implies f(x) = 2x( x - 5 ) ^2(x+4)^3`

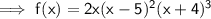
For finding the x intercept , equate the given function with 0, we have ;

`\sf \implies 2x ( x - 5 )^2(x+4)^3= 0`

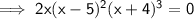
Equate each factor with 0 ,

`\sf \implies 2x = 0`

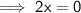
Divide both sides by 2 ,

`\sf \implies\bf x = 0`

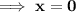
Again ,

`\sf \implies ( x - 5)^2=0`

Taking squareroot on both sides,

`\sf \implies x - 5 = 0`

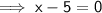
Add 5 to both sides,

`\sf \implies \bf x = 5`

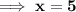
Similarly ,

`\sf \implies \bf x = -4`

Hence the x Intercepts are -4 , 0 and 5 .

{ See attachment also for graph } .