Question

Write a slope-intercept equation for a line perpendicular to f(x) = −2x + 4 and

passing through the point (−4, −1).

Answer 1

`y = (1)/(2) x + 1`

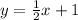
Explanation:

Slope-intercept form

y= mx +c, where m is the slope and c is the y-intercept

f(x)= -2x +4

Let's rewrite this function into an equation, in the slope-intercept form.

y= -2x +4

Slope= -2

The product of the slopes of perpendicular lines is -1.

Let the slope of the perpendicular line be m.

m(-2)= -1

m= ½

y= ½x +c

To find the value of c, substitute a pair of coordinates into the equation.

When x= -4, y= -1,

-1= ½(-4) +c

-1= -2 +c

c= -1 +2

c= 1

Thus, the equation of the line is y= ½x +1.