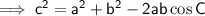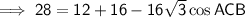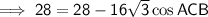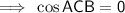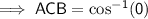Question

Cosine Rule In ABC, BC = 4cm, M is the mid-point of BC, AM = 4cm and AMB = 120..... Check the image below

Answer 1

`\sf (a) \quad AC=2√(3)\:\:cm`

`\sf (b) \quad AB=2√(7)\:\:cm`

`\sf (c) \quad \angle ACB=90^(\circ)`

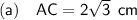
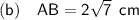
Explanation:

Cosine rule

`\sf c^2=a^2+b^2-2ab \cos C`

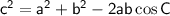
where:

• a, b and c are the sides of the triangle.
• C is the angle opposite side c.

Sketch the triangle using the given information (see attached).

Part (a)

Given:

• a = MC = 2
• b = AM = 4
• c = AC
• C = ∠AMC = 60°

Substitute the given values into the formula and solve for AC:

`\implies \sf c^2=a^2+b^2-2ab \cos C`

`\implies \sf AC^2=2^2+4^2-2(2)(4) \cos 60^(\circ)`

`\implies \sf AC^2=4+16-16 \left((1)/(2)\right)`

`\implies \sf AC^2=20-8`

`\implies \sf AC^2=12`

`\implies \sf AC=√(12)`

`\implies \sf AC=√(4 \cdot 3)`

`\implies \sf AC=√(4)√(3)`

`\implies \sf AC=2√(3)\:\:cm`

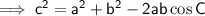
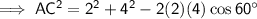
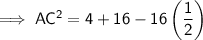
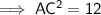
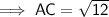
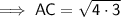
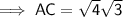
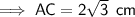
Part (b)

Given:

• a = BM = 2
• b = AM = 4
• c = AB
• C = ∠AMB = 120°

Substitute the given values into the formula and solve for AB:

`\implies \sf c^2=a^2+b^2-2ab \cos C`

`\implies \sf AB^2=2^2+4^2-2(2)(4) \cos 120^(\circ)`

`\implies \sf AB^2=4+16-16 \left(-(1)/(2)\right)`

`\implies \sf AB^2=20+8`

`\implies \sf AB^2=28`

`\implies \sf AB=√(28)`

`\implies \sf AB=√(4\cdot7)`

`\implies \sf AB=√(4)√(7)`

`\implies \sf AB=2√(7)\:\:cm`

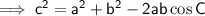
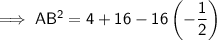
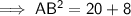
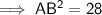
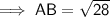
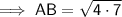
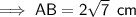
Part (c)

Given:

• a = AC = 2√3
• b = BC = 4
• c = AB = 2√7
• C = ∠ACB

Substitute the given values into the formula and solve for ∠ACB:

`\implies \sf c^2=a^2+b^2-2ab \cos C`

`\implies \sf \left(2√(7)\right)^2=\left(2√(3)\right)^2+4^2-2\left(2√(3)\right)(4) \cos ACB`

`\implies \sf 28=12+16-16√(3) \cos ACB`

`\implies \sf 28=28-16√(3) \cos ACB`

`\implies \sf 0=-16√(3) \cos ACB`

`\implies \sf \cos ACB=0`

`\implies \sf ACB=\cos^(-1)(0)`

`\implies \sf ACB=90^(\circ)`