Answer:
the first term
a₁ = -30.4
Explanation:
Let (an) be an arithmetic sequence with a common difference 8.
a₁ represents its first term.
And let S be the sum of the first 10 terms of the sequence.
Then
S = a₁ + a₂ + ………+ a₁₀
a₁₀ = a₁ + 8(10 - 1) = a₁ + 8×9 = a₁ + 72
S = a₁ + a₂ + ………+ a₁₀
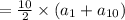
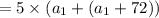


We are given : S = 56
then
56 = 10a₁ + 360
then
-10a₁ = 360 - 56
then
-10a₁ = 304
then
a₁ = -304/10
= -30.4