Question

What is the distance between (-5,0) and (0,3)

Answer 1

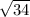`√(34)` or 5.83, approximately.

Explanation:

Let's refer to the formula for finding distances between points on a cartesian plane:

`D=\sqrt{(x_(2) -x_(1) )^(2)+{(y_(2) -y_(1) )^(2) }`

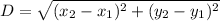
Let's solve the problem.

1. Identify all the variables for the formula.

In this case, we have 2 values of x, -5 and 0. x₁ would be -5, since it is the lesser value out of the 2. Therefore, x₂ is 0.

For the y values, now thatwe know that x₁ is -5, y₁ would be the number that -5 has on it's ordered pair, which is 0. y₂ is the last value that we have remaining to be assigned, 3. So, we have that:

x₁ = -5;

x₂ = 0;

y₁ = 0;

y₂ = 3.

2. Substitute the values in the formula.

`D=\sqrt{(x_(2) -x_(1) )^(2)+{(y_(2) -y_(1) )^(2) }\\\\`

`D=\sqrt{(0 -(-5) )^(2)+{(3 -0 )^(2)`

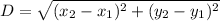
3. Simplify and calculate.

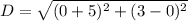`D=\sqrt{(0+5 )^(2)+{(3 -0 )^(2)` ----> Remember the laws of signs here. Minus and minus equals +.

`D=\sqrt{(5 )^(2)+{(3 )^(2)`

`D=\sqrt{25+{9`

`D=\sqrt{34`

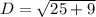
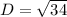
D≈5.83

4. Express a result.

You may let the result to be
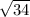`√(34)`, since it's a more accurate way of expressing it, but you can also say it's, approximately, 5.83.