Question

Find the distance between the two points.
(-5,1) and (-30,61)

Answer 1

65

Explanation:

You are going to create a right triangle between these two points. An ordered pair (x,y) can help us find the legs of the triangle. The difference in the x's will tell us the horizonal distance of one leg and the difference of the y's will tell us the vertical distance of the other leg. The slant that joins the two points completes our triangle and it is the hypotenuse.

(-5,1) (-30,61)

Let's look at the x values first. If I start at -5 and to to -30, what is the distance? 25 (distance is a positive number)

Let's look at the y values. If I start at 1 and go up to 61, what is the distance? 60

`a^(2) +b^(2) = c^(2)`

`25^(2) +60^(2) = c^(2)`

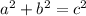
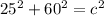
625 +3600 =
`c^(2)`

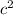
4225 =
`c^(2)`

`\sqrt{4225` =
`\sqrt{c^(2) }`

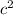
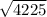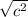
65 = c