Question

Find the center and radius of the circle whose equation is given by (-7)2(y+2)2-100.

Answer 1

Center = (7 , -2)

Radius = 10

Explanation:

To find the center of a circle in an equation. You always have to flip the value within the parenthesis. For example, in this case we have (x - 7) and (y + 2), flip the number to its opposite sign. So 7 would end up being positive and y would end up being negative. This makes our center.

(x - 7)^2 + (y + 2)^2 = 100

(7 , -2)

Now for radius, since the base equation for a circle is (x - h)^2 + (y - k)^2 = r^2. We have to take the square root of whatever is in r's place. Which in this case is 100. Do as said,

`√(100)`

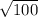
10

This makes 10 our radius.

Center = (7 , -2)

Radius = 10