Question

What is wrong with the following statement? If g/h = k, then g ∙ k = h.

Answer 1

k should be multiplied by h.

Explanation:

Answer 2

k should be multiplied by h

Explanation:

When working with fractions in algebra, it is important to remember how to cancel numerators and denominators.

Inaccuracies

The first statement given by the question is inaccurate. It is likely that this statement was obtained by multiplying both sides of
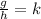`(g)/(h) =k` by g and then canceling out g on the left. However, this action would actually result in the new equation:

• 
  `\displaystyle(g^2)/(h) =kg`

The numerator does not cancel out when multiplying by itself. No matter how you manipulate this equation, the statement must be inaccurate. If you wanted to solve for h you would get
`(g)/(k) =h`.

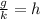Accurate Statment

One way to accurately manipulate this equation is to multiply by h (remember the multiplication property of equality). This looks like:

• 
  `\displaystyle(g)/(h) *(h)/(1) = kh`

The important part of this to note is that the h's cancel. Since the h is in the numerator of one fraction and the denominator of the other, you can cancel out the variable. The simplified version of this equation is:

• 
  `g=kh`