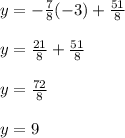Question

32. Find y if the line through (5, 2) and (-3. y ) has a slope of - 7/8​

Answer 1

y= 9.

Explanation:

• For this, we may use the formula to craft the linear equation for this point:

Fórmula:

`y-y_(1) =m(x-x_(1) )`

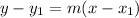
Substitute with the given values:

`y-2 =-(7)/(8) (x-5)`

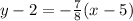
Simplify (make sure to apply the distributive property to multiply the slope by the 2 terms inside the parenthesis):

`y-2 =-(7)/(8) x+(35)/(8)`

Solve for y:

`y =-(7)/(8) x+(35)/(8)+2`

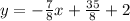
Simplify:

`y =-(7)/(8) x+(35)/(8)+(16)/(8) \\\\y =-(7)/(8) x+(51)/(8)`

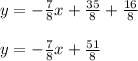
• Now that we have the equation of the line, take the knows values and solve calculate y:

`y =-(7)/(8) (-3)+(51)/(8)\\\\y =(21)/(8) +(51)/(8)\\\\y =(72)/(8)\\\\y=9`