Answer:
290 cm²
Explanation:
Area of regular hexagon-based pyramid.
First find the area of 6 triangles and then the area of the regular hexagon.
Slant height = s = 15 cm
side = b = 5 cm
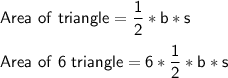
= 3 bs
= 3 * 5 * 15
= 225 cm²
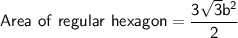

Area of regular hexagon based polygon = Area of 6 triangles + area of regular hexagon
= 225 + 64.95
= 289.95
= 290.0 cm²