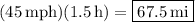Question

Two trains, Wand X are located 150 miles away from each other. Train W leaves Abbington bound for Belville, and train X leaves Belville bound for Abbington. If train W travels at 45 miles per hour and train X travels at 55 miles per hour what is the distance, in miles, that train W travels before they cross paths?

Answer 1

At time
`t` (in hours), train W travels a distance of

`(45\,\mathrm{mph})t`

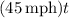
away from Abbington. Meanwhile, train X starts 150 mi away from Abbington and is getting closer, so its distance from Abbington is

`150\,\mathrm{mi} - (55\,\mathrm{mph})t`

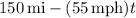
When the two trains meet, we have

`45t = 150 - 55t`

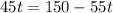
Solve for
`t`.

`45t = 150 - 55t \implies 100t = 150 \implies t = (150)/(100) = 1.5`

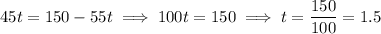
The trains pass each other after 1.5 hours, at which point train W will have traveled a distance of

`(45\,\mathrm{mph})(1.5\,\mathrm h) = \boxed{67.5\,\rm mi}`