Answer:
a.) V = √(mv²/M)
Explanation:
As with any "solve for ..." situation, you make use of inverse operations to "undo" what is done to the variable of interest.
Solve
In the given equation ...
MV² = mv²
the variable V is squared and that result is multiplied by M. These operations are dealt with in reverse order.
To undo multiplication by M, we divide both sides of the equation by M.
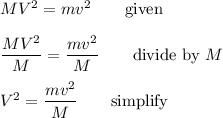
To undo the squaring of V, we take the square root.
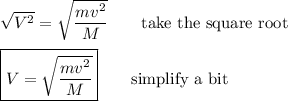
This matches the first choice.
__
Additional comment
Continued simplification would give you ...
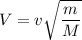
However, this is not one of the answer choices.