COMMON RATIO
ANSWER:
- The common ratio (r) is -0.5 or -½.
— — — — — — — — — —
In order to get the common ratio, divide any term of geometric sequence to its preceding term.
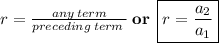
Substitute the given term and its preceding term to our equation, we get:
Thus, the answer in your question is -0.5 or -½.