18. Consider the two planes given by 3x - 6y - 2z = 3, 2x + y - 2z = 2 (a) The point (x, 0, 0) is on both planes. Find x. (b) Find a vector n normal to the first plane. (c) Find a vector n normal to the - QAmmunity.org

18. Consider the two planes given by 3x - 6y - 2z = 3, 2x + y - 2z = 2 (a) The point (x, 0, 0) is on both planes. Find x. (b) Find a vector n normal to the first plane. (c) Find a vector n normal to the

asked Sep 27, 2023 43.7k views

2 Answers

(a) If
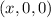(x,0,0) lies on both planes, then

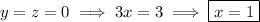
$y=z=0 \implies 3x = 3 \implies \boxed{x=1}$

and at the same time

$2x = 2 \implies x=1$

(b) A plane with normal vector
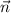$\vec n$ containing the point
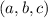(a,b,c) can be written in the form

$\vec n \cdot \langle x - a, y - b, z - c \rangle = 0$

Expanding the left side, we see that the components of
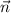$\vec n$ correspond to the coefficients of
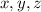x,y,z . So the normal vector to
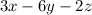3x-6y-2z is
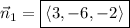$\vec n_1 = \boxed{\langle3,-6,-2\rangle}$ .

(c) Similarly, the normal to
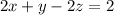2x+y-2z=2 is
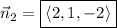$\vec n_2 = \boxed{\langle2,1,-2\rangle}$ .

(d) The cross product of any two vectors
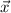$\vec x$ and
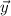$\vec y$ is perpendicular to both of the vectors. So we have

$\vec n_1 * \vec n_2 = \boxed{\langle 14, 2, 15\rangle}$

(e) Solve the two plane equations for
.

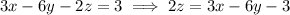
$3x - 6y - 2z = 3 \implies 2z = 3x - 6y - 3$

$2x + y - 2z = 2 \implies 2z = 2x + y - 2$

By substitution,

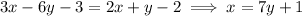
$3x - 6y - 3 = 2x + y - 2 \implies x = 7y + 1$

Let
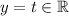$y=t\in\Bbb R$ . Then
x=7t+1 and

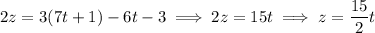
$2z = 3(7t+1) - 6t - 3 \implies 2z = 15t \implies z = \frac{15}2t$

Then the intersection can be parameterized by equations

$\begin{cases} x(t) = 7t + 1 \\\\ y(t) = t \\\\ z(t) = \frac{15}2 t \end{cases}$

for
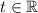$t\in\Bbb R$ .

We can also set
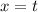x=t or
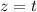z=t first, then solve for the other variables in terms of the parameter
, so this is by no means a unique parameterization.

Peter Schuetze answered Oct 4, 2023

by Peter Schuetze

7.1k points

Search QAmmunity.org