Answer:
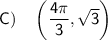
Explanation:
Given function:
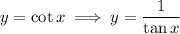
Substitute the x-values of the given coordinates into the given function:
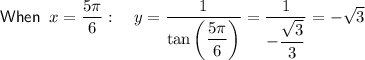
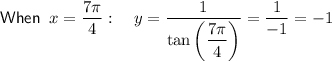
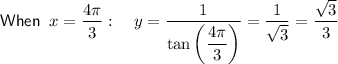
Comparing the y-values of the given coordinates, we can clearly see that point that is not on the graph of the function is:
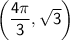
(Please see attached graph for proof).