Answer:
0
Explanation:
So we have the equation:

We can cross multiply to get the following equation:
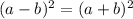
We can take the square root of both sides
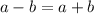
Add b and subtract a to both sides:
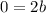
0 = b
If we plug in 0 as b
we get the following equation:
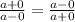
which is just:
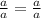
which is valid for all values except:
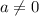
since b=0, then we get the equation:
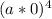 which is just:
which is just:
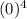 which is just 0
which is just 0
We took the positive solution, to the square root, but what if we had taken the negative solution?
Well we would've gotten the equation:
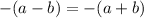
Distributing the signs we get:
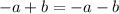
Add a to both sides
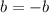
looking at this, it's obvious the only solution is 0, but we can also just add b to both sides
2b = 0
Now divide both sides by 2
b = 0
This gives us the same thing, and we can come to the same reasoning
The last thing to note is that, if a=0, then we have the fractions:
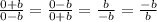
This equations are the exact same, since if we move the sign to the front we just get:

This works for all real numbers except when b=0
Since a=0 in all these cases, we get the equation:
(0 * b) ^ 4 = (0)^4 = 0