Answer:
Value of the car after x years
Explanation:
Exponential functions are generally expressed in the form:
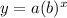 and since this function is decreasing, meaning it has an exponential decay, it can be expressed as:
and since this function is decreasing, meaning it has an exponential decay, it can be expressed as:
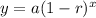 where r=rate of decay.
where r=rate of decay.
In this case the a represents the initial value or y-intercept, since when x=0, (1-r)^0 = 1, so we just have y=a(1) or y=a
The r represents the rate of decay
the x usually represents the time in seconds, days, months, or whatever unit is being used
The y value represents the value of whatever your measuring, and in this context it represents the value of the car after x years.
This is because a represents the initial value
After one year it's only 85% worth it's initial value or 15% less which is represented as 0.85(a)
After two years it's only 85% worth the previous year or 15% less the previous year, which is represented as 0.85(0.85(a))
This will continue to decrease the number of years increase