Question
If y = a + by + c(t)^2 where y = distance , t = time , find the dimension and unit of c.
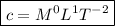
Solution Given:
We know that
Law of Homogeneity of Dimensions In any correct equation representing the relation between physical quantities, the dimensions of all the terms must be the same on both sides.
Dimension of Time is denoted by [T]
Dimension of distance is denoted by [L]
Dimension of mass is denoted by [M]
So,
Dimension of a, by, c(t)^2 should be equal to y.
y=[L] .......[1]
a=[L].......[2]
by=[L].......[3]
c(t)^2=[L].....[4]
Now
The dimension of c can be obtained as:
c(t)^2=[L]
c=
![([L])/([T^2])](https://img.qammunity.org/2023/formulas/physics/high-school/rt8kfciebuce1mc2bdrbojmxdya7ongqpa.png)
Sry for late!!