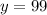Question

The sum of two numbers is 126. If three times the smaller number is subtracted from the larger​ number, the result is 18. Find the two numbers.

Answer 1

Larger number: 99

Smaller Number: 27

Explanation:

Ok, since both of these values are unknown, let's just say the smaller value is "x" and the bigger value is "y".

Since the sum of the two numbers is 126, then that means:

`x+y=126`

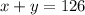
It also states "larger number - three times(smaller number) = 18" which it says in words, but is a bit confusing to comprehend, or maybe I'm slow.

Anyways, this gives us our second equation which is:

`y-3x=18`

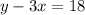
Remember, we assigned the smaller value to "x" and the bigger value to "y".

So now all we really have is a systems of equations, which we can easily solve by substitution. We can do this by solving for "y" or the larger number in the second equation.

Original Equation:

`y-3x=18`

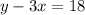
Add 3x to both sides

`y=3x+18`

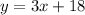
Now let's take a look at our other equation:

`x+y=126`

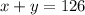
Since what we're looking for is for a x, y value pair that satisfies both equations, that must mean the "y" in our equation "y=3x+18" must equal the value of "y" in the our equation "x+y=126", meaning we can substitute "3x+18" as "y" in the equation "x+y=126"

`x+(3x+18)=126`

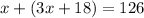
Add like terms:

`4x+18=126`

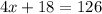
Subtract 18 from both sides

`4x=108`

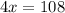
Divide both sides by 4

`x=27`

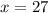
Now we can plug this into either equation to solve for "y", but the equation "x+y=126" is so much easier to solve for "y" if we know the value of "x", which we do.

`27+y=126\\`

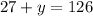
Subtract 27 from both sides

`y=99`