Answer:
○
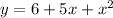
Step-by-step explanation:
What is a quadratic equation?
A quadratic equation is an algebraic equation where the highest power of
 is 2. The general form of a quadratic equation is as follows:
is 2. The general form of a quadratic equation is as follows:
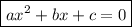 ,
,
where a, b, and c represent known constants, and
 is the unknown.
is the unknown.
In this question, if you take a look at the second option, you will see that it is possible to rearrange the equation to make it look the general form:
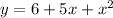
⇒
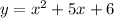
Therefore, this equation is quadratic.
The other three options are not quadratic equations because none of them have an
 term in them.
term in them.