The denominator cannot be zero, so

is not in the domain of
 .
.
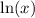 is defined only for
is defined only for
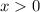 , and we have
, and we have
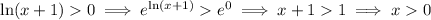
so there is no issue here.
By the same token, we need to have
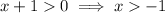
Taking all the exclusions together, we find the domain of
 is the set
is the set
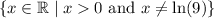
or equivalently, the interval
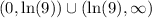 .
.