Question

2x³-8=0
How do I solve this problem ?

Answer 1

`x=2^{(2)/(3)}`

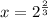
Explanation:

1) Add 8 to both sides.

`2x^3=8`

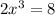
2) Divide both sides by 2.

`x^3=(8)/(2)`

3) Simplify
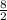`(8)/(2)` to 4.

`x^3=4`

4) Take the cube root of both sides.

`x=\sqrt[3]{4}`

5) Rewrite 4 as 2².

`x=\sqrt[3]{2^2}`

6) Use this rule:
`{({x}^(a))}^(b)={x}^(ab)`.

`x=2^{(2)/(3)}`

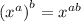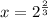
Decimal Form: 1.587401

__________________________________________

Check the answer:

`2x^3-8=0`

1) Let
`x=2^(2)/(3)`.

`2(2^{(2)/(3) })-8=0`

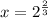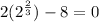
2) Use this rule:
`(x^a)^b=x^(ab)`.

`2*2^{(2*3)/(3) } -8=0`

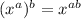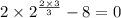
3) Simplify 2 * 3 to 6.

`2*2^{(6)/(3) } - 8 =0`

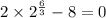
4) Simplify 6/3 to 2.

`2*2^2-8=0`

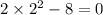
5) Use Product Rule:
`x^ax^b=x^(a+b)`.

`2^3-8=0`

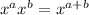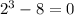
6) Simplify 2^3 to 8.

8 - 8 = 0

7) Simplify 8 - 8 to 0.

0 = 0

Thank you,

Eddie