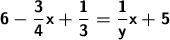
Convert 6 to the fraction 18/3.
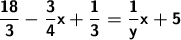
Since the fractions 18/3 and 1/3 have the same denominator, we add their numerators to calculate them.
![\boldsymbol{\sf{(18+1)/(3)-(3)/(4)x=(1)/(2)x+5 \ \longmapsto \ \ [Add \ 18+1] }}](https://img.qammunity.org/2023/formulas/mathematics/college/a1l1vc8av14aa2s1l2ogk949cbv65xfdbi.png)
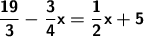
Subtract
 on both sides.
on both sides.
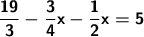
Combine
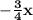 and
and
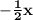 to get
to get
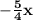 .
.
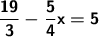
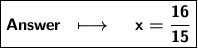
Subtract 19x from both sides.

Convert 5 to the fraction 15/3.
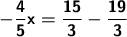
Since the fractions 15/3 and 19/3 have the same denominator, we add their numerators to calculate them.
![\boldsymbol{\sf{-(5)/(4)x=(15-19)/(3) \ \longmapsto \ \ [Subtract \ 15-19] }}](https://img.qammunity.org/2023/formulas/mathematics/college/zcr72s5gtjfbvaz0pm31x3mdzeg7248t9p.png)
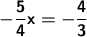
Multiply both sides by -4/3, the reciprocal of -4/3.
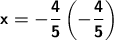
Multiply -4/3 by -4/5 (to do this, multiply the numerator by the numerator and the denominator by the denominator).