Question

To the nearest whole number, what is the surface area of the right triangular prism?

Answer 1

Answer: 797.4 m²

Explanation:

The surface area is just the total of the areas of each face of of a solid. In this solid, we have 2 triangles and 3 rectangles.

Triangles

We know that the two triangles of this solid are congruent, so they will have the same area. Since the area of a triangle is
`(1)/(2)bh`, two triangles would have an area of
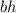`bh`. Hence, the total area is

`A=9 * 15\\A=135`

Rectangles

The area of a rectangle is lw, where l is the length and w is the width. Let's find the total area of all of them.

`A=9*16+15*16+17.4*16`

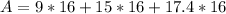
All of the areas are a product of some number and 16. This makes sense as the length of this prism is 16. We can un-distribute this 16 to make the calculation easier.

`A=16(9+15+17.4)\\A=16(41.4)\\A=662.4`

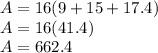
Total

We can add both totals to get the total surface area of the solid.

`135+662.4\\=797.4`

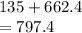
The surface area of this right triangular prism is 797.4 m².