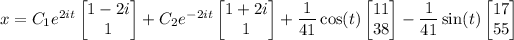It looks like the system is
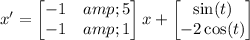
Compute the eigenvalues of the coefficient matrix.
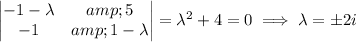
For
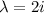 , the corresponding eigenvector is
, the corresponding eigenvector is
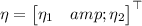 such that
such that
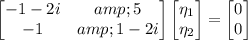
Notice that the first row is 1 + 2i times the second row, so
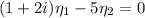
Let
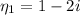 ; then
; then
 , so that
, so that
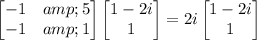
The eigenvector corresponding to
 is the complex conjugate of
is the complex conjugate of
 .
.
So, the characteristic solution to the homogeneous system is
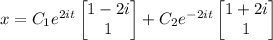
The characteristic solution contains
 and
and
 , both of which are linearly independent to
, both of which are linearly independent to
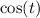 and
and
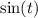 . So for the nonhomogeneous part, we consider the ansatz particular solution
. So for the nonhomogeneous part, we consider the ansatz particular solution
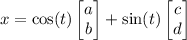
Differentiating this and substituting into the ODE system gives
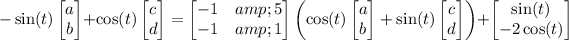
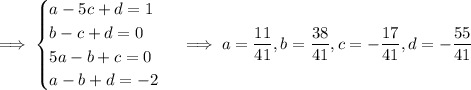
Then the general solution to the system is