Answer:
see explanation
Explanation:
(a)
A lies on the y- axis with x- coordinate of zero
substitute x = 0 into either of f(x) or g(x) for y- coordinate
g(0) = 2(0) + 1 = 0 + 1 = 1
then A (0, 1 )
---------------------
B and C lie on the x- axis with y- coordinate of zero
let f(x) = 0 and solve for x , that is
1 - x² = 0 , then
x² = 1 ( take square root of both sides )
x = ±
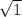 = ± 1
= ± 1
then B (- 1, 0 ) and C (1, 0 )
--------------------------------------
D is the point on the x- axis where g(x) crosses
let g(x) = 0 and solve for x
2x + 1 = 0 ( subtract 1 from both sides )
2x = - 1 ( divide both sides by 2 )
x = -

then D (-
 , 0 )
, 0 )
-----------------------------
(b)
E is the point of intersection of f(x) and g(x) so equate them, that is
2x + 1 = 1 - x² ( subtract 1 - x² from both sides )
x² + 2x = 0 ← factor out x from each term
x(x + 2) = 0
equate each factor to zero and solve for x
x = 0 ← x- coordinate of A
x + 2 = 0 ⇒ x = - 2 ← x- coordinate of E
substitute x = - 2 into g(x) for corresponding y- coordinate
g(- 2) = 2(- 2) + 1 = - 4 + 1 = - 3
then E (- 2, - 3 )