Question

Law of cosines

HELP MES

Answer 1

70.52 degrees

Explanation:

To find the angles, we must first find the lengths of each side of the triangle. Adding up the respective radii, we can see that

XY = 5+4 = 9 CM

XZ = 6+5 = 11 CM

ZY = 4+6 = 10 CM

Now we can apply the cosine rule

`C\:=\:√(A^2+B^2-2ABcosx)`

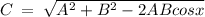
We need to rearrange the rule to solve for x, our missing angle

`x\:=\:cos^(-1)\left((a^2+b^2-c^2)/(2ab)\right)`

solving for our unknown angle:

`x\:=\:cos^(-1)\left((9^2+10^2-11^2)/(2\cdot 9\cdot 10)\right)`

`x\:=\:70.52^(\circ )`

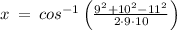
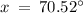
Therefore angle YXZ is 70.52 degrees