Question

Can someone help me with a step by step process of how to solve this? Calculus 2

Answer 1

If you fix a point on the curve in the given interval, and revolve that point about the
`x`-axis, it will trace out a circle with radius given by the function value
`y` for that point
`x`. The perimeter of this circle is then
`2\pi(8\sqrt x) = 16\pi \sqrt x`.

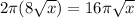The surface in question is essentially what you get by joining infinitely many of these circles at every point in the interval [0, 9].

So, the surface area is given by the definite integral

`\displaystyle \int_0^9 16\pi \sqrt x \, dx = 16\pi*\frac23 x^(3/2)\bigg|_(x=0)^(x=9) = \frac{32\pi}3 \left(9^(3/2) - 0^(3/2)\right) = \boxed{288\pi}`