Answer:
9.2195445 (9.22 rounded)
Explanation:
#1: Use the distance formula to determine the distance between the two points:
Distance =
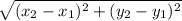
#2: Substitute the actual values of the points into the distance formula:
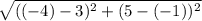
#3: Simplify:
- Subtract 3 from -4:
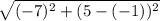
- Raise -7 to the power of 2:
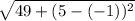
- Multiply -1 by -1:
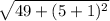
- Add 5 and 1:

- Raise 6 to the power of 2:
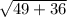
- Add 49 and 36:
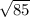 (Decimal form: 9.2195445 (9.22 rounded))
(Decimal form: 9.2195445 (9.22 rounded))
The distance between the two points (3,1) and (4,-5) is 9.22.