Question

Someone please help meeeeeee

Answer 1

a ≈ 16.5 cm , b ≈ 23.8 cm

Explanation:

using the Law of Sines in Δ ABC

`(a)/(sinA)` =
`(b)/(sinB)` =
`(c)/(sinC)`

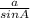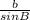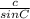
we require to calculate ∠ C

∠ C = 180° - (42 + 75)° = 180° - 117° = 63°

Then to find a

`(a)/(sinA)` =
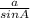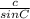`(c)/(sinC)` ( substitute values )

`(a)/(sin42)` =
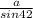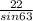`(22)/(sin63)` ( cross- multiply )

a × sin63° = 22 × sin42° ( divide both sides by sin63° )

a =
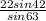`(22sin42)/(sin63)` ≈ 16.5 cm ( to the nearest tenth )

similarly to find b

`(b)/(sinB)` =
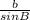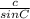`(c)/(sinC)` ( substitute values )

`(b)/(sin75)` =
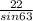`(22)/(sin63)` ( cross- multiply )

b × sin63° = 22 × sin75° ( divide both sides by sin63° )

b =
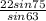`(22sin75)/(sin63)` ≈ 23.8 cm ( to the nearest tenth )

Answer 2

Explanation:

Sine rule of Law of sine:

`\sf \boxed{\bf(a)/(Sin \ A)=(b)/(Sin \ B)=(c)/(Sin \ C)}`

Side 'a' faces ∠A.

Side 'b' faces ∠B.

Side 'c' faces ∠C.

We have to find ∠C using angle sum property of triangle.

∠C + 75 + 42 = 180

∠C +117 = 180

∠C = 180 - 117

∠C = 63°

`\sf (a)/(Sin \ 42)= (22)/(Sin \ 63)\\\\ (a)/(0.67)=(22)/(0.89)\\\\`

`\sf a = (22)/(0.89)*0.67\\\\ \boxed{a = 16.56 \ cm }`

`\sf (b)/(Sin \ B) = (c)/(Sin \ C)\\\\ (b)/(Sin \ 75)=(22)/(Sin 63)\\\\ (b)/(0.97) =(22)/(0.89)\\\\`

`\sf b = (22)/(0.89)*0.97\\\\ \boxed{b =23.98 \ cm }`