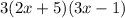Answer:
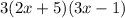
Explanation:
Hello!
We can first factor out the greatest common factor between the coefficients: 3.
Now, let's work with what we have inside the brackets.
Standard form of a quadratic:

Given our equation:

We need to find two numbers that add up to b (13) and multiply to ac (-30).
The two numbers are 15 and -2. Expand 13x to 15x and -2x and factor by grouping.
Factor by Grouping
Now, we simply add the other factor, 3, to the final factored form.
Factored Solution: