Question

The steps to derive the quadratic formula are shown below:

Step 1 ax2 + bx + c = 0
Step 2 ax2 + bx = − c
Step 3 x2 + b over a times x equals negative c over a
Step 4


Provide the next step to derive the quadratic formula.
x squared plus b over a times x minus quantity b over 2 times a all squared equals negative c over a minus quantity b over 2 times a all squared
x squared plus b over a times x plus quantity b over 2 times a all squared equals negative c over a plus quantity b over 2 times a all squared
x squared plus b over a times x minus quantity 2 times a over b all squared equals negative c over a minus quantity 2 times a over b all squared
x squared plus b over a times x plus quantity 2 times a over b all squared equals negative c over a plus quantity 2 times a over b all squared

Answer 1

Below in bold.

Explanation:

The next step is to divide b/a by 2 then square it and add to both sides.

This creates a perfect square quadratic on left side.

So the answer is :

x squared plus b over a times x plus quantity b over 2 times a all squared equals negative c over a plus quantity b over 2 times a all squared

Answer 2

`\huge\boxed{\sf Option \ B}`

Explanation:

Step 3:

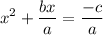`\displaystyle x^2+(bx)/(a) =(-c)/(a)` --------------------(1)

The next step will be:

• to find the b² for the expression on the left.

How to find b²:

Take the expression

`\displaystyle x^2 + (bx)/(a)`

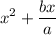
We can also write it as:

`\displaystyle (x)^2 + 2(x)((b)/(2a) )`

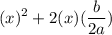
According to the formula
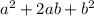`a^2+2ab+b^2`, the b of this expression is
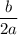`\displaystyle (b)/(2a)`. So,

b² will be:

`\displaystyle =((b)/(2a) )^2\\\\=(b^2)/(4a^2)`

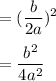
So, we will add
`\displaystyle (b^2)/(4a^2)` to both sides in Eq. (1)

For STEP 4, the equation will become:

`\displaystyle x^2+(bx)/(a) + (b^2)/(4a^2) = (-c)/(a) + (b^2)/(4a^2)`

`\rule[225]{225}{2}`