Question

The function f(x) = 200(0.5)x/50 models the amount in pounds of a particular

radioactive material stored in a concrete vault, where x is the number of years since
the material was put into the vault. Find the amount of radioactive material in the
vault after 140 years. Round to the nearest whole number.

Answer 1

29 years

Explanation:

• Given the function
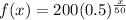  `f(x)= 200(0.5)^{(x)/(50) }`, where x stands for the number of years since the material was put into the vault, we can plug 140 into x to find the amount leftover.
• Another way of doing this is to change the function to
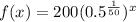  `f(x)=200(0.5^{(1)/(50) })^(x)` which are equivalent functions, and then plug 140 into x. This function is helpful if you also needed to find the rate.
• I'll use the 1st function because the question doesn't ask for the rate

`f(x)= 200(0.5)^(140)/(50)}`

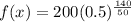
From here I'll just use a calculator & get 28.7, or 29 years.