The sum of three numbers is 78. the third number is 3 times the first. the first number is 7 more than the second. what are the numbers?

Question

asked Feb 27, 2023 111k views

2 Answers

Amir Nissim · Answer 1 · 2023-03-05T00:41:09+0000

Answer:

The three numbers are 17, 10 and 51.

Explanation:

Let the first, second, and third numbers be
,
, and
respectively.

• From the question, we know:

sum of the numbers is 78.

∴
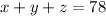x + y + z = 78 ----------(1st equation)

Let's express both
and
in terms of
$\bf y$ :

• We know that:

the third number is 3 times the first.

∴
z = 3x

⇒
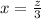x = (z)/(3) ----------(2nd equation)

• We also know that:

the first number is 7 more than the second.

∴
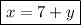
$\boxed{x = 7 + y}$

Substituting
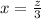x = (z)/(3) (from 2nd equation)

⇒
(z)/(3) = 7 + y

⇒
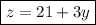
$\boxed{z = 21 + 3y}$

• We can now substitute
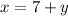x = 7 + y and
z = 21 + 3y into the first equation:

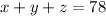
x + y + z = 78

⇒
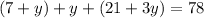
(7 + y) + y + (21 + 3y) = 78

⇒
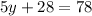
5y + 28 = 78

⇒
5y = 50

⇒
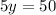
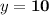
$y = \bf 10$

∴
$x = 7 + 10\\$

⇒
$x = \bf 17$

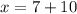
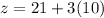
z = 21 + 3(10)

⇒
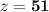
$z = \bf 51$

∴ The three numbers are 17, 10 and 51.