Answer:
w= 9
Explanation:
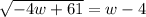
Square both sides:
-4w +61= (w -4)²
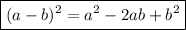
Expand:
-4w +61= w² -2(w)(4) +4²
-4w +61= w² -8w +16
Simplify:
w² -8w +16 +4w -61= 0
w² -4w -45= 0
Factorize:
(w -9)(w +5)= 0
w -9= 0 or w +5= 0
w= 9 or w= -5 (reject)
Note:
-5 is rejected since we are only taking the positive value of the square root here. If the negative square root is taken into consideration, then w= -5 would give us -9 on both sides of the equation.
Why do we use negative square root?
When solving an equation such as x²= 4,
we have to consider than squaring any number removes the negative sign i.e., the result of a squared number is always positive.
In the case of x²= 4, x can be 2 or -2. Thus, whenever we introduce a square root, a '±' must be used.
However, back to our question, we did not introduce the square root so we should not consider the negative square root value.