Determining the slopes of each side, we get the slope of
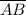 is
is
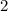 , the slope of
, the slope of
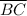 is -1/2, the slope of
is -1/2, the slope of
 is 2, and the slope of
is 2, and the slope of
 is -1/2. Since the slopes of sides AB and CD and BC and AD are equal, it follows that
is -1/2. Since the slopes of sides AB and CD and BC and AD are equal, it follows that
 and
and
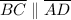 . Thus, ABCD is a parallelogram because it is a quadrilateral with two pairs of opposite congruent sides. However, we can also note that the slope of side AB is the negative reciprocal of that of sides BC, and thus
. Thus, ABCD is a parallelogram because it is a quadrilateral with two pairs of opposite congruent sides. However, we can also note that the slope of side AB is the negative reciprocal of that of sides BC, and thus
 . Using the fact that perpendicular lines form right angles, we can conclude that
. Using the fact that perpendicular lines form right angles, we can conclude that
 is a right angle, and since ABCD is thus a parallelogram with a right angle, it must also be a rectangle.
is a right angle, and since ABCD is thus a parallelogram with a right angle, it must also be a rectangle.