Question

Which side lengths form a right triangle?

Choose all answers that apply:
A:
11,13,√290
B:
7, 24, 25
C: 2, 5, 7

Answer 1

Answer: A, B

Explanation:

A right triangle's sides have a special property that the sum of the squares of the two legs is equal to the square of the hypotenuse. This is also known as the Pythagorean Theorem.

Let's check if this theorem holds true for each triangle.

A

`11^2+13^2=(√(290))^2\\ 121 + 169=290\\ 290 = 290`

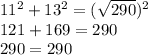
The Pythagorean Theorem holds true, so this is a right triangle.

B

`7^2+24^2=25^2\\ 49 + 576 = 625\\ 625=625`

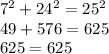
The Pythagorean Theorem holds true, so this is a right triangle.

C

`2^2+5^2=7^2\\ 4+25=49\\ 29 = 49`

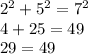
This is a false statement, which doesn't make this triangle a right triangle. We could have use the triangle inequality theorem to prove that this isn't a triangle at all, and therefore not a right triangle, but I encourage you to learn about it and try it yourself.

Answer 2

B

Explanation:

Use the Pythagorean theorem, 7^2+24^2=25^2

49+576=625

625=625

All the other answers do not satisfy the Pythagorean theorem (a^2+b^2=c^2)