Answer:
 or 2.738
or 2.738
Explanation:
Let’s just look at the triangle on the top with the
 on the top and x on the bottom. (Basically the top half to the equilateral triangle)
on the top and x on the bottom. (Basically the top half to the equilateral triangle)
There is a small square in the bottom right corner, which indicates that this triangle is a right triangle. This means that we can use the Pythagorean Theorem:
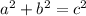
We know that \sqrt{10} is our hypotenuse, and therefore our c in our equation. Let’s say that x=a in our equation. Therefore we are left to find b. However, b is half the length of the side of the original equilateral triangle. An equilateral triangle means that all three sides are the same length. Therefore our side would also be \sqrt{10} units long. However we know that b is half of that value, so b=
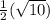 or
or
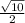
Plugging these values into the equation:
x^2+ (\frac{\sqrt{10} }{2})^{2}=\sqrt{10} ^{2}
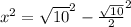

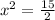
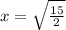
This approximately equals 2.738